ACM
July 20, 2021

数位 DP

数位 DP

简介

给定区间 [L, R]，求该区间中符合某种条件的数的个数

HDU 2089 · 不要 62

Description

给定两个数 L, R，计算 [L, R] 中数位上没有出现 4 或 62（ 连续）的数的个数

Solution

  1. 求符合条件的数的个数

    1
    2
    3
    4
    5
    6
    7
    8
    9
    10
    11
    12
    13
    14
    15
    16
    17
    18
    19
    20
    int dp[10][10];	//dp[x][y]：长度为 x，首位为 y 的数中符合条件的个数
    dp[0][0] = 1;
    for (int l = 1; l <= 7; l++)
    {
        for (int i = 0; i <= 9; i++)
        {
            if (i == 4) dp[l][i] = 0;
            else
            {
            	for (int j = 0; j <= 9; j++)
            	{
                	if (i == 6 && j == 2) continue;
                    else
                    {
                        dp[l][i] += dp[l - 1][j];
                    }
            	}
            }
        }
    }

  2. 求 [0, n] 中符合条件的数的个数

    设 n = 234

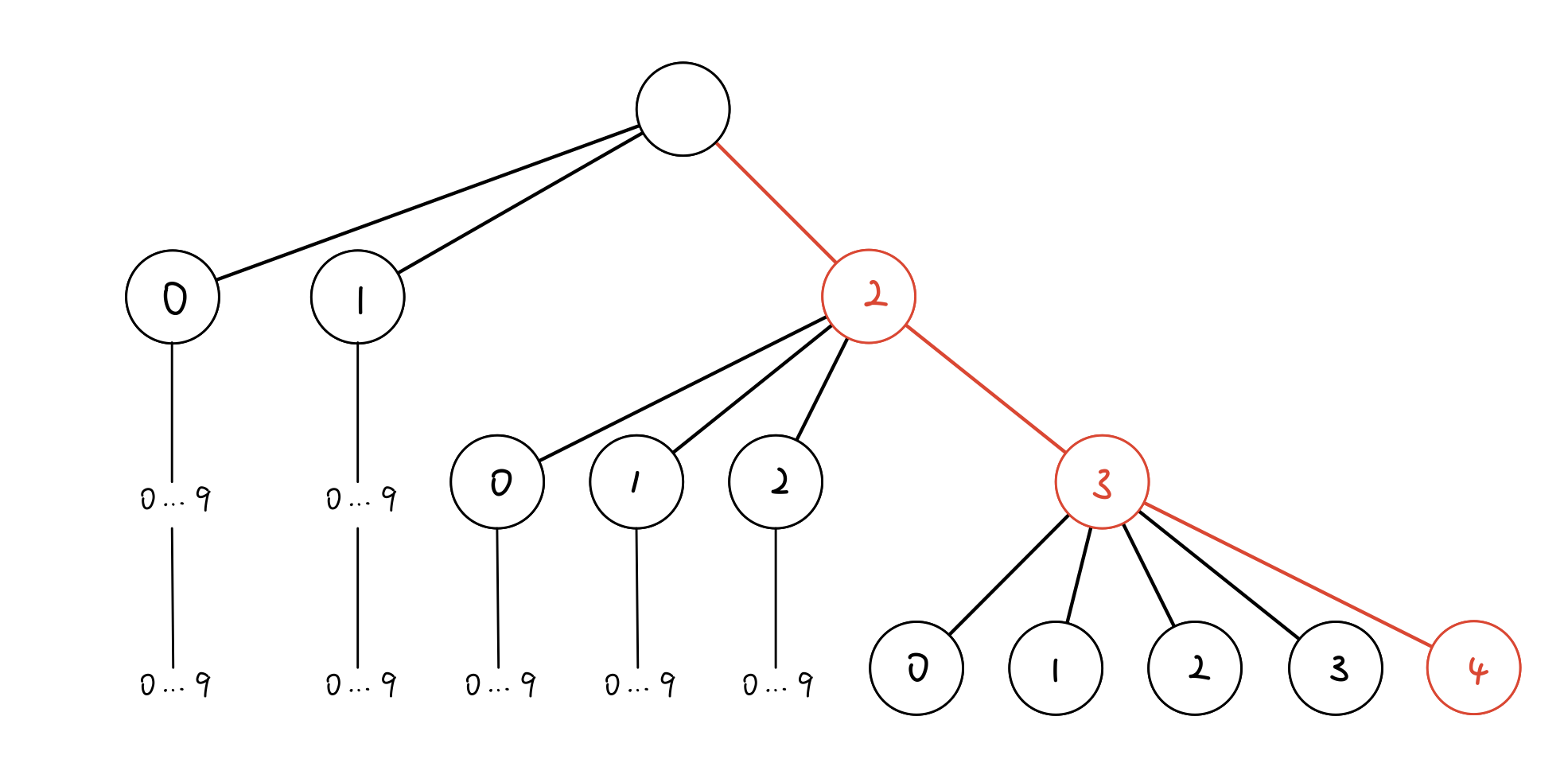
    显然答案不是 dp[3][2]，因为三位数以 2 开头的数中包含 235 等大于 234 的数

    因此要按如下分段求：

    • dp[3][0] + dp[3][1] 求出三位数中 0xx, 1xx
    • dp[2][0] + dp[2][1] + dp[2][2] 求出两位数中 0x, 1x, 2x
    • dp[1][0] + dp[1][1] + dp[1][2] + dp[1][3] 求出一位数中 0, 1, 2, 3

    但是假如分段中含 4 或者 62 的，如 345，则遇到 4 或 62 就停止，因为

    继续细分出的段的高位肯定包含了 4 或 62

    • dp[3][0] + dp[3][1] + dp[3][2] 求出三位数中 0xx, 1xx, 2xx
    • dp[2][0] + dp[2][1] + dp[2][2] + dp[2][3] 求出两位数中 0x, 1x, 2x, 3x

Accepted Code

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
#define _CRTSECURE_NOWARNINGS
#pragma warning(disable:4996)
#include <cstdio>
#include <cstring>
#include <string>
#include <cmath>
#include <cctype>
#include <algorithm>
#include <iostream>
#include <queue>
#include <stack>
#include <vector>
#include <map>
#include <set>
#include <list>
using namespace std;

int m, n;
int dp[10][10];
int d[10];

void Init()
{
	dp[0][0] = 1;
	for (int l = 1; l <= 7; l++)
	{
		for (int i = 0; i <= 9; i++)
		{
			if (i == 4) dp[l][i] = 0;
			else
			{
				for (int j = 0; j <= 9; j++)
				{
					if (i == 6 && j == 2) continue;
					else
					{
						dp[l][i] += dp[l - 1][j];
					}
				}
			}
		}
	}
}

int get(int n)
{
	int ans = 0;
	int len = 0;
	while (n)
	{
		++len;
		d[len] = n % 10;
		n /= 10;
	}
	d[len + 1] = 0;
	for (int i = len; i >= 1; --i)
	{
		for (int j = 0; j < d[i]; ++j)
		{
			if (d[i + 1] != 6 || j != 2)
				ans += dp[i][j];
		}
		if (d[i] == 4 || (d[i + 1] == 6 && d[i] == 2))
			break;
	}
	return ans;
}

int main()
{
	Init();
    
	while (1)
	{
		scanf("%d%d", &m, &n);
		if (!n && !m) return 0;
		printf("%d\n", get(n + 1) - get(m));
	}
    
    
	return 0;
    
}

SCOI 2009 · Windy 数

Description

给定一个区间 [L, R]，求其中满足条件不含前导 0 且相邻两个数字相差至少为 2 的数字个数。

Accepted Code

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
#define _CRTSECURE_NOWARNINGS
#pragma warning(disable:4996)
#include <cstdio>
#include <cstring>
#include <string>
#include <cmath>
#include <cctype>
#include <algorithm>
#include <iostream>
#include <queue>
#include <stack>
#include <vector>
#include <map>
#include <set>
#include <list>
using namespace std;

int dp[11][11];
int a[11], p, q;

void Init()
{
	for (int i = 0; i <= 9; i++) dp[1][i] = 1;
	for (int l = 2; l <= 10; l++)
	{
		for (int i = 0; i <= 9; i++)
		{
			for (int j = 0; j <= 9; j++)
			{
				if (abs(i - j) >= 2) dp[l][i] += dp[l - 1][j];
			}
		}
	}
}

int work(int x)	//计算 <=x 的 windy 数 
{
	memset(a, 0, sizeof(a));
	int len = 0, ans = 0;
	while (x)
	{
		a[++len] = x % 10;
		x /= 10;
	}
	//分为几个板块 先求 len - 1 位的 windy 数 必定包含在区间里的 
	for (int i = 1; i <= len - 1; i++)
	{
		for (int j = 1; j <= 9; j++)
		{
			ans += dp[i][j];
		}
	}
	//然后是 len 位 但最高位 < a[len] 的 windy 数 也包含在区间里 
	for (int i = 1; i < a[len]; i++)
	{
		ans += dp[len][i];
	}
	//接着是 len 位 最高位与原数相同的 最难搞的一部分 
	for (int i = len - 1; i >= 1; i--)
	{
		//i 从最高位后开始枚举 
		for (int j = 0; j <= a[i] - 1; j++)
		{
			//j 是 i 位上的数 
			if (abs(j - a[i + 1]) >= 2)	ans += dp[i][j]; //判断和上一位 (i + 1) 相差 2 以上
			   //如果是 ans 就累加 
		}
		if (abs(a[i + 1] - a[i]) < 2) break;
		//  if(i == 1)   ans += 1;
	}
	return ans;
}

int main()
{
	Init();
	cin >> p >> q;
	cout << work(q + 1) - work(p) << endl;
	return 0;
}

About this Post

This post is written by OwlllOvO, licensed under CC BY-NC 4.0.

#C++#数位 DP